Proposition: 3.07: Relative Lengths of Lines Inside Circle

(Proposition 7 from Book 3 of Euclid's “Elements”)

If some point, which is not the center of the circle, is taken on the diameter of a circle, and some straight lines radiate from the point towards the (circumference of the) circle, then the greatest (straight line) will be that on which the center (lies), and the least the remainder (of the same diameter). And for the others, a (straight line) nearer¹ to the (straight line) through the center is always greater than a (straight line) further away. And only two equal (straight lines) will radiate from the point towards the (circumference of the) circle, (one) on each (side) of the least (straight line).

Let $ABCD$ be a circle, and let $AD$ be its diameter, and let some point $F$, which is not the center of the circle, have been taken on $AD$.
Let $E$ be the center of the circle.
And let some straight lines, $FB$, $FC$, and $FG$, radiate from $F$ towards (the circumference of) circle $ABCD$.
I say that $FA$ is the greatest (straight line), $FD$ the least, and of the others, $FB$ (is) greater than $FC$, and $FC$ than $FG$.

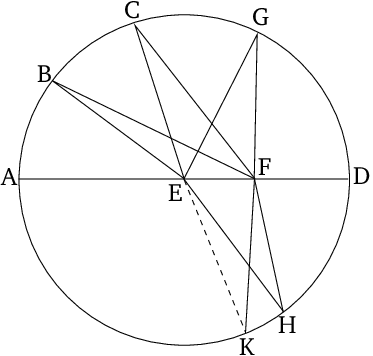
fig07e

Modern Formulation

If a point $F$ is located on the diameter ($\overline{AD}$) of a given circle and is not its center ($E$), then if we connect this point $F$ to all points of the circumference, we get a infinite family of segments, among which exactly one is the longest (let it be $\overline{FA}$ with the length $\beta$), exactly one is the shortest (let it be $\overline{FD}$ with the length $\alpha$) and all other (infinitely many) segments are located on both sides of the diameter, and their lengths $\gamma$ take all values between $ \alpha\le \gamma\le \beta$. Moreover, among these infinitely many segments there are exactly two segments with the same length $\gamma$, namely those which lie symmetrically with respect to the diameter $\overline{AD}.$

Proofs: 1

Thank you to the contributors under CC BY-SA 4.0!