Proposition: 6.03: Angle Bisector Theorem

(Proposition 3 from Book 6 of Euclid's “Elements”)

If an angle of a triangle is cut in half, and the straight line cutting the angle also cuts the base, then the segments of the base will have the same ratio as the remaining sides of the triangle. And if the segments of the base have the same ratio as the remaining sides of the triangle, then the straight line joining the vertex to the cutting (point) will cut the angle of the triangle in half.

Let $ABC$ be a triangle.
And let the angle $BAC$ have been cut in half by the straight line $AD$.
I say that as $BD$ is to $CD$, so $BA$ (is) to $AC$.

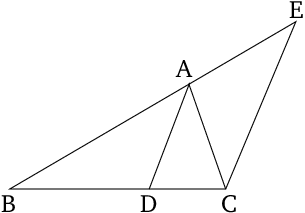
fig03e

Modern Formulation

Let a straight line $AD$ cut the base $BC$ of a triangle $\bigtriangleup{ABC}$ at the point $D.$ Then the straight line $AD$ bisects the angle $\angle{BAC}$ if and only if the lengths of the cut subsements of the base ($\overline{BD},\overline{DC}$) are proportional to the lengths of the remaining sides ($\overline{AB},\overline{AC}$) of the triangle: $$\frac{|\overline{BD}|}{|\overline{DC}|}=\frac{|\overline{AB}|}{|\overline{AC}|}.$$

Proofs: 1

Thank you to the contributors under CC BY-SA 4.0!