Proposition: 6.11: Construction of Segment in Squared Ratio

(Proposition 11 from Book 6 of Euclid's “Elements”)

To find a third (straight line) proportional to two given straight lines.

Let $BA$ and $AC$ be the [two] given [straight lines], and let them be laid down encompassing a random angle.
So it is required to find a third (straight line) proportional to $BA$ and $AC$.

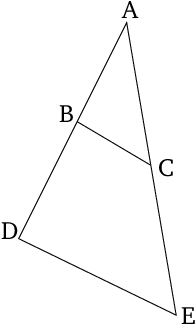
fig11e

Modern Formulation

Given two segments $\overline{AB}$, $\overline{AC}$ with the ratio $$\frac{|\overline{AB}|}{|\overline{AC}|},$$ it is possible to construct a third segment $\overline{CE}$ with a squared ratio. $$\frac{|\overline{AB}|}{|\overline{AC}|}=\frac{|\overline{AC}|}{|\overline{CE}|}.$$

This construction can be repeated $2$ times to construct a segment with a cubed ratio or an arbitrary number $n$ of times to construct a segment with the $n-1$-th continued proportion.

Proofs: 1

Proofs: 1 2

Thank you to the contributors under CC BY-SA 4.0!