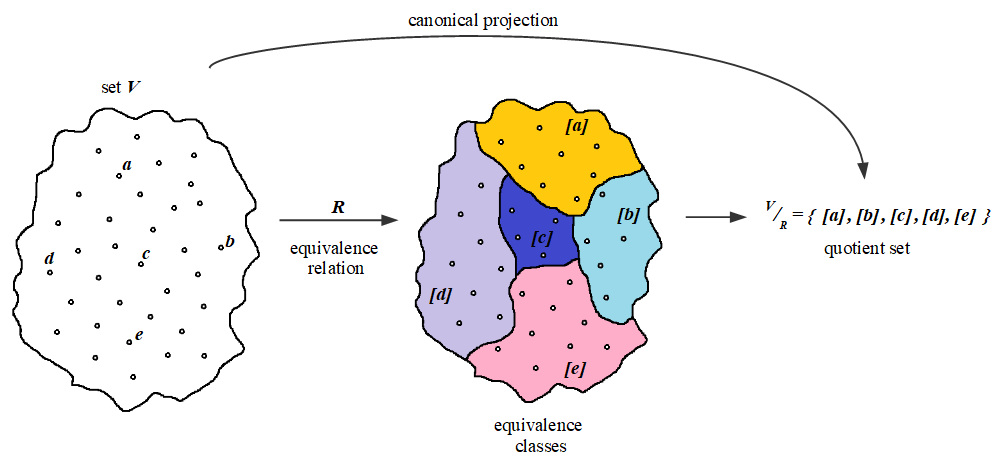
Although the idea of a function will be introduced later, we anticipate it here already and mention a special kind of functions which naturally occur along with equivalence relations: the canonical projections.
Let \(V\) be a set, \(R\subseteq V\times V\) an equivalence relation and let \(V/_R\) be the corresponding quotient set. The function $q\colon V\rightarrow V/_R,\,a\mapsto [a]\,$ is called the canonical projection of \(R\).