I have a set of four dice, not marked with spots in an ordinary way, but with Arabic figures, as shown in the illustration.
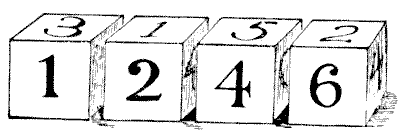
Each die, of course, bears the numbers $1$ to $6.$ When put together, they will form a good many, different numbers. As represented they make the number $1246.$ Now, if I make all the different four-figure numbers that are possible with these dice (never putting the same figure more than once in any number), what will they all add up to? You are allowed to turn the $6$ upside down, so as to represent a $9.$ I do not ask, or expect, the reader to go to all the labor of writing out the full list of numbers and then adding them up. Life is not long enough for such wasted energy. Can you get at the answer in any other way?
Solutions: 1
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.