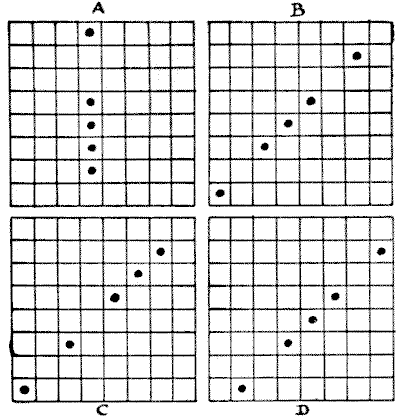
(related to Problem: The Five Dogs Puzzle)
The diagrams show four fundamentally different solutions. In the case of $A$ we can reverse the order so that the single dog is in the bottom row and the other four shifted up two squares. Also, we may use the next column to the right and both of the two central horizontal rows. Thus $A$ gives $8$ solutions. Then B may be reversed and placed in either diagonal, giving $4$ solutions. Similarly, $C$ will give $4$ solutions. The line in $D$ being symmetrical, its reversal will not be different, but it may be disposed in $4$ different directions. We thus have in all $20$ different solutions.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.