(related to Problem: Painting A Pyramid)
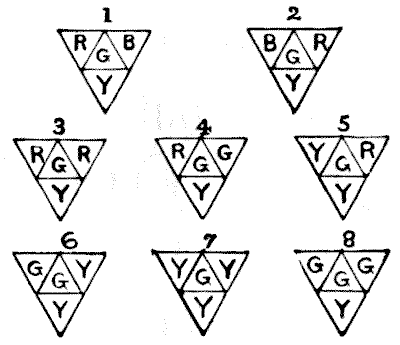
It will be convenient to imagine that we are painting our pyramids on the flat cardboard, as in the diagrams, before folding up. Now, if we take any four colors (say red, blue, green, and yellow), they may be applied in only $2$ distinctive ways, as shown in Figs, $1$ and $2.$ Any other way will only result in one of these when the pyramids are folded up. If we take any three colors, they may be applied in the $3$ ways shown in Figs. $3,$ $4,$ and $5.$ If we take any two colors, they may be applied in the $3$ ways shown in Figs. $6,$ $7,$ and $8.$ If we take any single color, it may obviously be applied in only $1$ way. But four colors may be selected in $35$ ways out of seven; three in $35$ ways; two in $21$ ways; and one color in $7$ ways. Therefore $35$ applied in $2$ ways $= 70;$ $35$ in $3$ ways $= 105;$ $21$ in $3$ ways $= 63;$ and $7$ in $1$ way$= 7.$ Consequently, the pyramid may be painted in $245$ different ways $(70 + 105 + 63 + 7),$ using the seven colors of the solar spectrum in accordance with the conditions of the puzzle.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.