(related to Problem: Two Crosses From One)
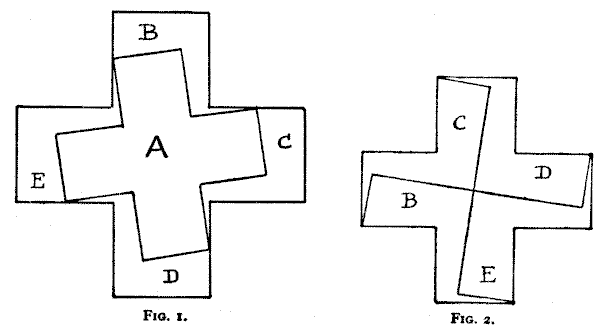
It will be seen that one cross is cut out entire, as $A$ in Fig. $1,$ while the four pieces marked $B, C, D$ and $E$ form the second cross, as in Fig. $2,$ which will be of exactly the same size as the other. I will leave the reader the pleasant task of discovering for himself the best way of finding the direction of the cuts. Note that the Swastika again appears.
The difficult question now presents itself: How are we to cut three Greek crosses from one in the fewest possible pieces? As a matter of fact, this problem may be solved in as few as thirteen pieces; but as I know many of my readers, advanced geometricians, will be glad to have something to work on of which they are not shown the solution, I leave the mystery for the present undisclosed.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.