(related to Problem: Mrs. Smiley's Christmas Present)
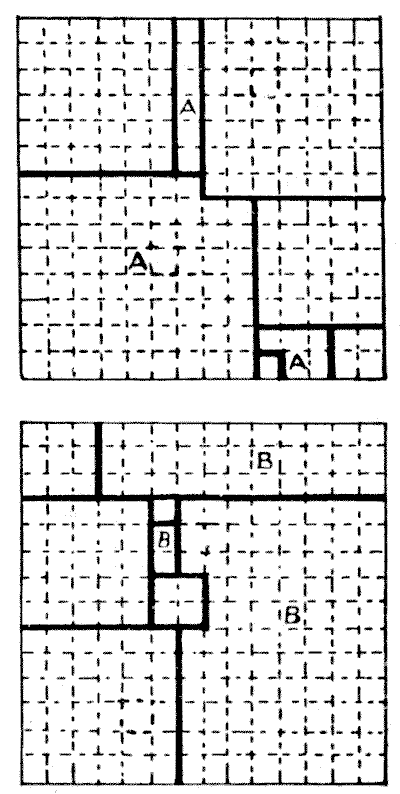
The first step is to find six different square numbers that sum to $196.$ For example, $$\begin{array}{rcl}1 + 4 + 25 + 36 + 49 + 81 &=& 196\\1 + 4 + 9 + 25 + 36 + 121 &=& 196\\1 + 9+ 16 + 25 + 64 + 81 &=& 196.\end{array}$$ The rest calls for individual judgment and ingenuity, and no definite rules can be given for procedure. The annexed diagrams will show solutions for the first two cases stated. Of course, the three pieces marked $A$ and those marked $B$ will fit together and form a square in each case. The assembling of the parts may be slightly varied, and the reader may be interested in finding a solution for the third set of squares I have given.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.