Proposition: 3.36: Tangent Secant Theorem

(Proposition 36 from Book 3 of Euclid's “Elements”)

If some point is taken outside a circle, and two straight lines radiate from it towards the circle, and (one) of them cuts the circle, and the (other) touches (it), then the (rectangle contained) by the whole (straight line) cutting (the circle), and the (part of it) cut off outside (the circle), between the point and the convex circumference, will be equal to the square on the tangent (line).

For let some point $D$ have been taken outside circle $ABC$, and let two straight lines, $DC[A]$ and $DB$, radiate from $D$ towards circle $ABC$.
And let $DCA$ cut circle $ABC$, and let $BD$ touch (it).
I say that the rectangle contained by $AD$ and $DC$ is equal to the square on $DB$.

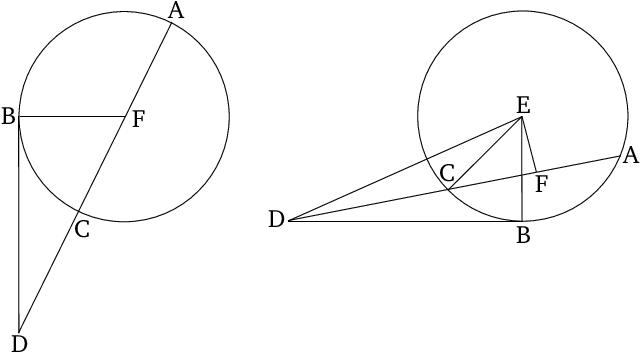
fig36e

Modern Formulation

Let, from a point $D$ outside a given circle, a tangent $DB$ touching the circle at $D$ be drawn and let a secant go through $D$ and cut this circle at the points $C$ and $A$ such that for the lengths segments the inequality $|\overline{DC}|<|\overline{DA}|$ holds. Then $$|\overline{DC}|\cdot |\overline{DA}|=|\overline{DB}|^2.$$

Proofs: 1

Proofs: 1
Sections: 2

Thank you to the contributors under CC BY-SA 4.0!