Proposition: 6.14: Characterization of Congruent Parallelograms

(Proposition 14 from Book 6 of Euclid's “Elements”)

In equal and equiangular parallelograms the sides about the equal angles are reciprocally proportional. And those equiangular parallelograms in which the sides about the equal angles are reciprocally proportional are equal.

Let $AB$ and $BC$ be equal and equiangular parallelograms having the angles at $B$ equal.
And let $DB$ and $BE$ be laid down straight-on (with respect to one another).
Thus, $FB$ and $BG$ are also straight-on (with respect to one another) [Prop. 1.14].
I say that the sides of $AB$ and $BC$ about the equal angles are reciprocally proportional, that is to say, that as $DB$ is to $BE$, so $GB$ (is) to $BF$.

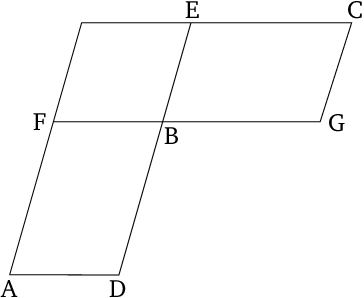
fig14e

Modern Formulation

Two parallelograms are congruent if and only if their angles and the products¹ of the side lengths of an angle are in both paralleograms equal.

$$\begin{array}{rclc} \boxdot{ADBF}\cong\boxdot{BCEG}&\Longleftrightarrow&(\angle{ADB}=\angle{BGC})&\wedge\\ &&(\angle{FAD}=\angle{EBG})&\wedge\\ &&(|\overline{DB}|\cdot|\overline{BF}|=|\overline{BE}|\cdot|\overline{GB}|). \end{array}$$

Proofs: 1

Proofs: 1 2 3 4 5 6

Thank you to the contributors under CC BY-SA 4.0!

Github:
non-Github:: @Fitzpatrick

References

Adapted from (subject to copyright, with kind permission)

Fitzpatrick, Richard: Euclid's "Elements of Geometry"

Adapted from CC BY-SA 3.0 Sources:

Prime.mover and others: "Pr∞fWiki", https://proofwiki.org/wiki/Main_Page, 2016

Footnotes

The product is equivalent to Euclid's "reciprocal proportion" $$\frac{|\overline{DB}|}{|\overline{BE}|}=\frac{|\overline{GB}|}{|\overline{BF}|},$$ which is not to be confused with the concept of reciprocal ratio. ↩

◀ ▲ ▶Branches / Geometry / Elements-euclid / Book--6-similar-figures / Proposition: 6.14: Characterization of Congruent Parallelograms