Proposition: Prop. 10.086: Construction of Second Apotome

To find a second apotome.

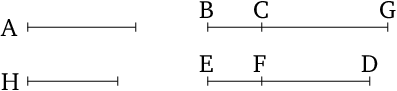
Let the rational (straight line) $A$ , and $GC$ (which is) commensurable in length with $A$ , be laid down.
Thus, $GC$ is a rational (straight line).
And let the two square numbers $DE$ and $EF$ be laid down, and let their difference $DF$ be not square [Prop. 10.28 lem. I] .
And let it have been contrived that as $FD$ (is) to $DE$ , so the square on $CG$ (is) to the square on $GB$ [Prop. 10.6 corr.] .
Thus, the square on $CG$ is commensurable with the square on $GB$ [Prop. 10.6].
And the (square) on $CG$ (is) rational.
Thus, the (square) on $GB$ [is] also rational.
Thus, $BG$ is a rational (straight line).
And since the square on $GC$ does not have to the (square) on $GB$ the ratio which (some) square number (has) to (some) square number, $CG$ is incommensurable in length with $GB$ [Prop. 10.9].
And they are both rational (straight lines).
Thus, $CG$ and $GB$ are rational (straight lines which are) commensurable in square only.
Thus, $BC$ is an apotome [Prop. 10.73].
So, I say that it is also a second (apotome) .