This is quite a fascinating little puzzle. Place eight bishops (four black and four white) on the reduced chessboard, as shown in the illustration.
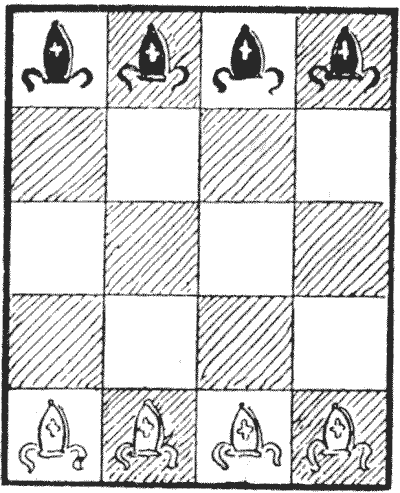
The problem is to make the black bishops change places with the white ones, no bishop ever attacking another of the opposite color.
They must move alternately — first a white, then a black, then a white, and so on. When you have succeeded in doing it at all, try to find the fewest possible moves. If you leave out the bishops standing on black squares and only play on the white squares, you will discover my last puzzle turned on its side.
Solutions: 1
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.