(related to Problem: A Cutting-out Puzzle)
The below illustration shows how to cut the four pieces and form with them a square. First, find the side of the square (the mean proportional between the length and height of the rectangle), and the method is obvious. If our strip is exactly in the proportions $9\times1,$ or $16x\times1,$ or $25\times1,$ we can clearly cut it in $3,$ $4,$ or $5$ rectangular pieces respectively to form a square. Excluding these special cases, the general law is that for a strip in length more than $n^2$ times the breadth, and not more than $(n+1)^2$ times the breadth, it may be cut in $n+2$ pieces to form a square, and there will be $n-1$ rectangular pieces like piece $4$ in the diagram.
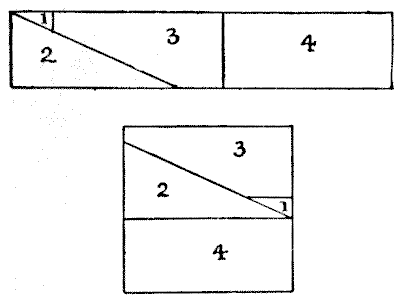
Thus, for example, with a strip $24\times1,$ the length is more than $16$ and less than $25$ times the breadth. Therefore it can be done in $6$ pieces ($n$ here being $4$), $3$ of which will be rectangular. In the case where $n$ equals $1,$ the rectangle disappears and we get a solution in three pieces. Within these limits, of course, the sides need not be rational: the solution is purely geometrical.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.