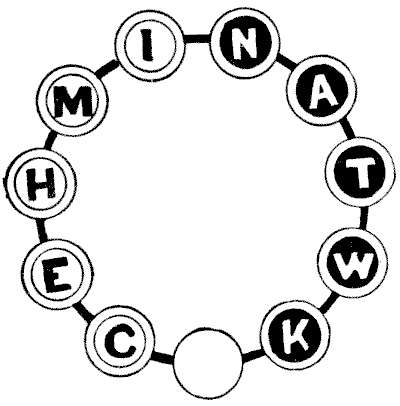
In the illustration we have eleven discs in a circle. On five of the discs we place white counters with black letters — as shown — and on five other discs the black counters with white letters. The bottom disc is left vacant. Starting thus, it is required to get the counters in order so that they spell the word "Twickenham" in a clockwise direction, leaving the vacant disc in the original position.
The black counters move in the direction that a clock-hand revolves, and the white counters go the opposite way.
A counter may jump over another counter of the opposite color if the vacant disc is next beyond. Thus, if your first move is with $K,$ then $C$ can jump over $K.$ If then $K$ moves towards $E,$ you may next jump $W$ over $C,$ and so on. The puzzle may be solved in twenty-six moves. Remember a counter cannot jump over its own color.
Solutions: 1
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.