(related to Problem: The Domino Frame Puzzle)
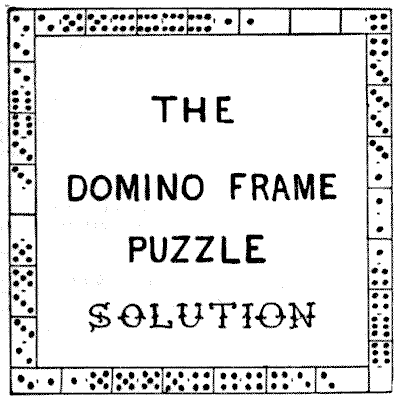
The illustration is a solution. It will be found that all four sides of the frame add up $44.$ The sum of the pips on all the dominoes is $168,$ and if we wish to make the sides sum to $44,$ we must take care that the four corners sum to $8,$ because these corners are counted twice, and $168$ added to $8$ will equal $4$ times $44,$ which is necessary. There are many different solutions. Even in the example given certain interchanges are possible to produce different arrangements. For example, on the left-hand side the string of dominoes from $2—2$ down to $3—2$ may be reversed, or from $2—6$ to $3—2,$ or from $3—0$ to $5—3.$ Also, on the right-hand side, we may reverse from $4—3$ to $1—4.$ These changes will not affect the correctness of the solution.
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.