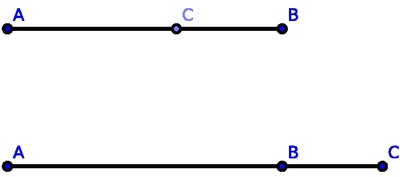
If a point \(C\) is taken on a segment \(\overline{AB}\), point \(C\) is called the point of division between segments \(\overline{AC}\) and \(\overline{CB}\). We also say that the segment \(\overline{AB}\) is divided at the point \(C\).
If the segment \(\overline{AB}\) is extended to point \(C\), then point \(C\) is called a point of external division.