◀ ▲ ▶Branches / Geometry / Elements-euclid / Book--4-inscription-and-circumscription / Definition: 4.4: Circumscribing Rectilinear Figures about Circles

Definition: 4.4: Circumscribing Rectilinear Figures about Circles

(Definitions 4 from Book 4 of Euclid's “Elements”)

And a rectilinear figure is said to be circumscribed about a circle when each side of the circumscribed (figure) touches the circumference of the circle.

Modern Formulation

A rectilinear figure (\(n\)-sided figure, \(n \ge 3\)) is said to be circumscribed about a circle when each side of the figure is tangent to the circumference of the circle.

Example

The pentagon is circumscribed about a circle:

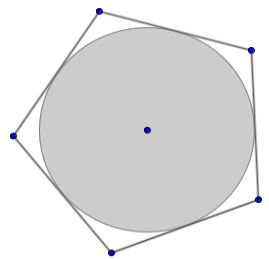
Mentioned in:

Corollaries: 1
Definitions: 2
Proofs: 3 4 5 6 7
Propositions: 8 9 10

Thank you to the contributors under CC BY-SA 4.0!

Github:

non-Github:

@Fitzpatrick

References

Bibliography

1. Byrne, O.: "The First Six Books of the Elements of Euclid, in Which Coloured Diagrams and Symbols are used Instead of Letters", London William Pickering, 1847

Adapted from (subject to copyright, with kind permission)

1. Fitzpatrick, Richard: Euclid's "Elements of Geometry"

Adapted from CC BY-SA 3.0 Sources:

1. Prime.mover and others: "Pr∞fWiki", https://proofwiki.org/wiki/Main_Page, 2016