◀ ▲ ▶Branches / Geometry / Elements-euclid / Book-10-incommensurable-magnitudes / Proof: By Euclid

Proof: By Euclid

(related to Proposition: Prop. 10.018: Condition for Incommensurability of Roots of Quadratic Equation)

• Let $A$ and $BC$ be two unequal straight lines, of which (let) $BC$ (be) the greater.
• And let a (rectangle) equal to the fourth [part] of the (square) on the lesser, $A$, falling short by a square figure, have been applied to $BC$.
• And let it be the (rectangle contained) by $BDC$.
• And let $BD$ be incommensurable in length with $DC$.
• I say that the square on $BC$ is greater than the (square on) $A$ by the (square) on (some straight line) incommensurable (in length) with ($BC$).

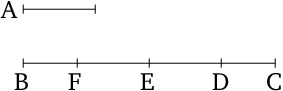
• For, similarly, by the same construction as before, we can show that the square on $BC$ is greater than the (square on) $A$ by the (square) on $FD$.
• Therefore, it must be shown that $BC$ is incommensurable in length with $DF$.
• For since $BD$ is incommensurable in length with $DC$, $BC$ is thus also incommensurable in length with $CD$ [Prop. 10.16].
• But, $DC$ is commensurable (in length) with the sum of $BF$ and $DC$ [Prop. 10.6].
• And, thus, $BC$ is incommensurable (in length) with the sum of $BF$ and $DC$ [Prop. 10.13].
• Hence, $BC$ is also incommensurable in length with the remainder $FD$ [Prop. 10.16].
• And the square on $BC$ is greater than the (square on) $A$ by the (square) on $FD$.
• Thus, the square on $BC$ is greater than the (square on) $A$ by the (square) on (some straight line) incommensurable (in length) with ($BC$).
• So, again, let the square on $BC$ be greater than the (square on) $A$ by the (square) on (some straight line) incommensurable (in length) with ($BC$).
• And let a (rectangle) equal to the fourth [part] of the (square) on $A$, falling short by a square figure, have been applied to $BC$.
• And let it be the (rectangle contained) by $BD$ and $DC$.
• It must be shown that $BD$ is incommensurable in length with $DC$.
• For, similarly, by the same construction, we can show that the square on $BC$ is greater than the (square) on $A$ by the (square) on $FD$.
• But, the square on $BC$ is greater than the (square) on $A$ by the (square) on (some straight line) incommensurable (in length) with ($BC$).
• Thus, $BC$ is incommensurable in length with $FD$.
• Hence, $BC$ is also incommensurable (in length) with the remaining sum of $BF$ and $DC$ [Prop. 10.16].
• But, the sum of $BF$ and $DC$ is commensurable in length with $DC$ [Prop. 10.6].
• Thus, $BC$ is also incommensurable in length with $DC$ [Prop. 10.13].
• Hence, via separation, $BD$ is also incommensurable in length with $DC$ [Prop. 10.16].
• Thus, if there are two ... straight lines, and so on ....
  ∎

Thank you to the contributors under CC BY-SA 4.0!

Github:

non-Github:

@Fitzpatrick

References

Adapted from (subject to copyright, with kind permission)

1. Fitzpatrick, Richard: Euclid's "Elements of Geometry"