◀ ▲ ▶Branches / Number-systems-arithmetics / Proposition: Discovery of Irrational Numbers

Proposition: Discovery of Irrational Numbers

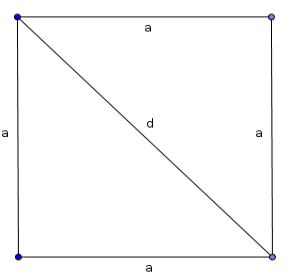
The side \(a\) and the diagonal \(d\) in any square are incommensurable. \[d=\sqrt{2}\cdot a.\]

In particular and for \(a=1\), there are no positive integers \(q,p\) such that \[q\cdot\sqrt 2=p\quad\text{or}\quad\sqrt 2=\frac pq.\]

Table of Contents

Proofs: 1 2

Mentioned in:

Definitions: 1
Parts: 2
Proofs: 3

Thank you to the contributors under CC BY-SA 4.0!

Github:

References

Bibliography

1. Alsina, Claudi; Nelsen, Roger B.: "Bezaubernde Beweise", Springer Spektrum, 2013