(related to Proposition: Time Dilation, Lorentz Factor)
Let \(\mathcal I_1\) and \(\mathcal I_2\) be two inertial frames of reference. Assume that a light clock is installed in \(\mathcal I_2\) with mirrors at a distance \(d_2\). An observer \(o_2\), who is situated in \(\mathcal I_2\), will observe a tick duration of \(t_2={d_2}/c\).
Assume that \(\mathcal I_1\) and \(\mathcal I_2\) are moving relatively to each other with the constant speed \(v\) and that the light clock is positioned perpendicular to the direction of this movement.
From the perspective of another observer \(o_1\) situated in \(\mathcal I_1\), the light clock in \(\mathcal I_2\) will cover the distance \(x\) within the time of one "tick" of the light clock. Let the duration of this "tick" be \(t_1\) from the perspective of the observer \(o_1\). Note that from the perspective of the observer \(o_1\) the light will cover the distance \(d_1\), which is given by hypotenuse the right triangle \(\triangle {ABC}\), as shown in the following figure:
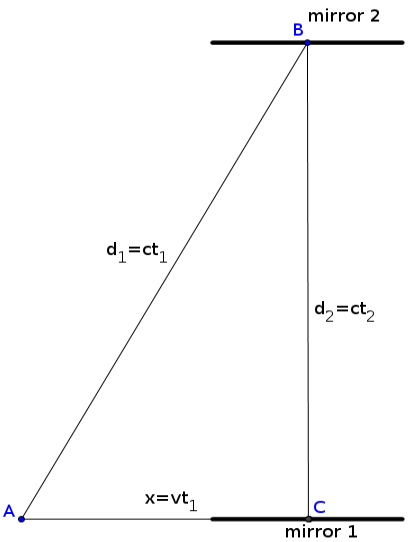
(c) bookofproofs own work
According to the Pythagorean theorem, we get \[d_1^2=x^2+d_2^2,\] which is equivalent to
\[\begin{array}{rcl}&&(ct_1)^2=(vt_1)^2+(ct_2)^2\\ &\Leftrightarrow&c^2t_1^2=v^2t_1^2+c^2t_2^2\\ &\Leftrightarrow&c^2t_1^2-v^2t_1^2=c^2t_2^2\\ &\Leftrightarrow&t_1^2-\frac{v^2}{c^2}t_1^2=t_2^2\\ &\Leftrightarrow&t_1^2\left(1-\frac{v^2}{c^2}\right)=t_2^2.\\ \end{array}\quad\quad ( * )\]
By hypothesis, \(0\le v < c\), from which it follows \[0\le \frac{v^2}{c^2} < 1\] and \[1\ge 1- \frac{v^2}{c^2} > 0.\quad\] Because the term \(1- v^2/c^2\) is non negative, is possible to calculate the square root, leading to the inequation \[1\ge \sqrt{1- v^2/c^2} > 0.\quad\quad ( * * )\]
It follows together with \(( * )\) that
\[t_1\sqrt{\left(1-\frac{v^2}{c^2}\right)}=t_2.\]
Because \(( * * )\) is non zero, we can solve the equation for \(t_1\) leading to the equation
\[t_1=t_2\frac 1{\sqrt{\left(1-\frac{v^2}{c^2}\right)}}.\quad\quad(0\le v < c)\]