A curve \(f:I\to\mathbb R^n.\) is called simple or a Jordan arc, if it is injective, i.e. if for any two \(x,y\in I\) from \(f(x)=f(y)\) it follows that \(x=y\).
Please note that the injectivity of \(f\) assures that there are no two points \(x,y\) with \(x\neq y\), for which \(f(x)=f(y)\). In other words, the curve has no "intersection points". For a curve in the plane \(\mathbb R^2\) this can be visualized as follows:
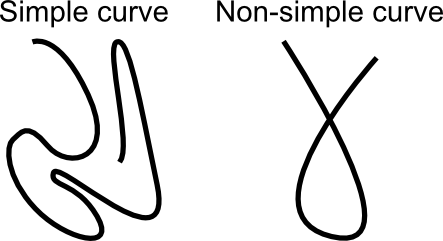
(from http:mathinsight.org)