I happened to have a number of strips of cardboard lying on my table, with numbers printed on them from $1$ upwards in numerical order. The idea suddenly came to me, as ideas have a way of unexpectedly coming, to make a little puzzle of this. I wonder whether many readers will arrive at the same solution that I did.
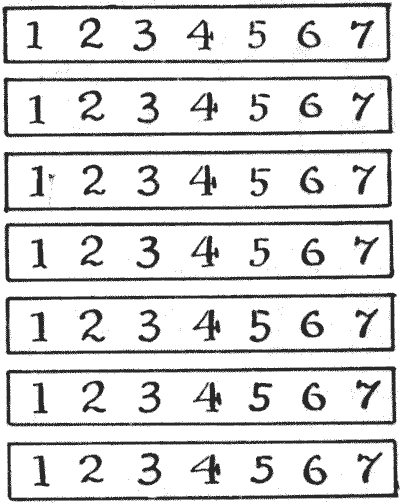
Take seven strips of cardboard and lay them together as above. Then write on each of them the numbers $1, 2, 3, 4, 5, 6, 7,$ as shown, so that the numbers shall form seven rows and seven columns.
Now, the puzzle is to cut these strips into the fewest possible pieces so that they may be placed together and form a magic square, the seven rows, seven columns, and two diagonals adding up the same number. No figures may be turned upside down or placed on their sides—that is, all the strips must lie in their original direction.
Of course, you could cut each strip into seven separate pieces, each piece containing a number, and the puzzle would then be very easy, but I need hardly say that forty-nine pieces are a long way from being the fewest possible.
Solutions: 1
This eBook is for the use of anyone anywhere in the United States and most other parts of the world at no cost and with almost no restrictions whatsoever. You may copy it, give it away or re-use it under the terms of the Project Gutenberg License included with this edition or online at http://www.gutenberg.org. If you are not located in the United States, you'll have to check the laws of the country where you are located before using this ebook.