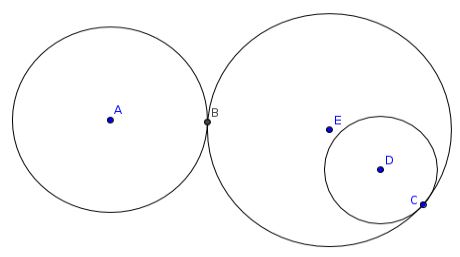
Circles said to touch one another are any (circles) which, meeting one another, do not cut one another.
Circles are said to touch one another when they intersect at exactly one point. There are two types of contact: 1. When a circle \(\odot AB\) is external to another circle \(\odot EB\), we say that the circle \(\odot AB\) touches the circle \(\odot EB\) externally at the point \(B\). 1. When one circle \(\odot DC\) is internal to another circle \(\odot EC\), we say that the circle \(\odot DC\) touches the circle \(\odot EC\) internally at the point \(C\).
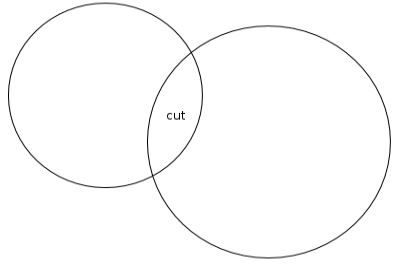
When circles intersect at two points, the intersection may be referred to as a cut.
Proofs: 1 2 3 4 5
Propositions: 6 7 8 9 10 11