◀ ▲ ▶Branches / Geometry / Elements-euclid / Book-13-platonic-solids / Proof: By Euclid

Proof: By Euclid

(related to Proposition: Prop. 13.08: Straight Lines Subtending Two Consecutive Angles in Regular Pentagon cut in Extreme and Mean Ratio)

• For let the two straight lines, $AC$ and $BE$, cutting one another at point $H$, have subtended two consecutive angles, at $A$ and $B$ (respectively), of the equilateral and equiangular pentagon $ABCDE$.
• I say that each of them has been cut in extreme and mean ratio at point $H$, and that their greater pieces are equal to the sides of the pentagon.

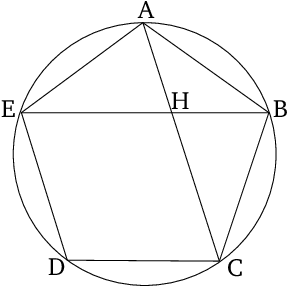
• For let the circle $ABCDE$ have been circumscribed about pentagon $ABCDE$ [Prop. 4.14].
• And since the two straight lines $EA$ and $AB$ are equal to the two (straight lines) $AB$ and $BC$ (respectively), and they contain equal angles, the base $BE$ is thus equal to the base $AC$, and triangle $ABE$ is equal to triangle $ABC$, and the remaining angles will be equal to the remaining angles, respectively, which the equal sides subtend [Prop. 1.4].
• Thus, angle $BAC$ is equal to (angle) $ABE$.
• Thus, (angle) $AHE$ (is) double (angle) $BAH$ [Prop. 1.32].
• And $EAC$ is also double $BAC$, inasmuch as circumference $EDC$ is also double circumference $CB$ [Prop. 3.28], [Prop. 6.33].
• Thus, angle $HAE$ (is) equal to (angle) $AHE$.
• Hence, straight line $HE$ is also equal to (straight line) $EA$ - that is to say, to (straight line) $AB$ [Prop. 1.6].
• And since straight line $BA$ is equal to $AE$, angle $ABE$ is also equal to $AEB$ [Prop. 1.5].
• But, $ABE$ was shown (to be) equal to $BAH$.
• Thus, $BEA$ is also equal to $BAH$.
• And (angle) $ABE$ is common to the two triangles $ABE$ and $ABH$.
• Thus, the remaining angle $BAE$ is equal to the remaining (angle) $AHB$ [Prop. 1.32].
• Thus, triangle $ABE$ is equiangular to triangle $ABH$.
• Thus, proportionally, as $EB$ is to $BA$, so $AB$ (is) to $BH$ [Prop. 6.4].
• And $BA$ (is) equal to $EH$.
• Thus, as $BE$ (is) to $EH$, so $EH$ (is) to $HB$.
• And $BE$ (is) greater than $EH$.
• $EH$ (is) thus also greater than $HB$ [Prop. 5.14].
• Thus, $BE$ has been cut in extreme and mean ratio at $H$, and the greater piece $HE$ is equal to the side of the pentagon.
• So, similarly, we can show that $AC$ has also been cut in extreme and mean ratio at $H$, and that its greater piece $CH$ is equal to the side of the pentagon.
• (Which is) the very thing it was required to show.
  ∎

Thank you to the contributors under CC BY-SA 4.0!

Github:

non-Github:

@Fitzpatrick

References

Adapted from (subject to copyright, with kind permission)

1. Fitzpatrick, Richard: Euclid's "Elements of Geometry"