Proof: By Euclid
(related to Proposition: Prop. 13.02: Converse of Area of Square on Greater Segment of Straight Line cut in Extreme and Mean Ratio)
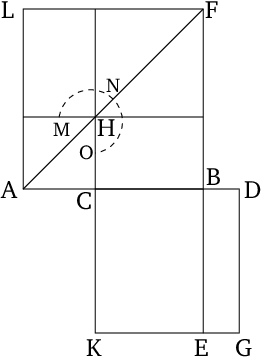
- For let the squares $AF$ and $CG$ have been described on each of $AB$ and $CD$ (respectively).
- And let the figure in $AF$ have been drawn.
- And let $BE$ have been drawn across.
- And since the (square) on $BA$ is five times the (square) on $AC$, $AF$ is five times $AH$.
- Thus, gnomon $MNO$ (is) four times $AH$.
- And since $DC$ is double $CA$, the (square) on $DC$ is thus four times the (square) on $CA$ - that is to say, $CG$ (is four times) $AH$.
- And the gnomon $MNO$ was also shown (to be) four times $AH$.
- Thus, gnomon $MNO$ (is) equal to $CG$.
- And since $DC$ is double $CA$, and $DC$ (is) equal to $CK$, and $AC$ to $CH$, [$KC$ (is) thus also double $CH$], (and) $KB$ (is) also double $BH$ [Prop. 6.1].
- And $LH$ plus $HB$ is also double $HB$ [Prop. 1.43].
- Thus, $KB$ (is) equal to $LH$ plus $HB$.
- And the whole gnomon $MNO$ was also shown (to be) equal to the whole of $CG$.
- Thus, the remainder $HF$ is also equal to (the remainder) $BG$.
- And $BG$ is the (rectangle contained) by $CDB$.
- For $CD$ (is) equal to $DG$.
- And $HF$ (is) the square on $CB$.
- Thus, the (rectangle contained) by $CDB$ is equal to the (square) on $CB$.
- Thus, as $DC$ is to $CB$, so $CB$ (is) to $BD$ [Prop. 6.17].
- And $DC$ (is) greater than $CB$ (see lemma).
- Thus, $CB$ (is) also greater than $BD$ [Prop. 5.14].
- Thus, if the straight line $CD$ is cut in extreme and mean ratio then the greater piece is $CB$.
- Thus, if the square on a straight line is five times the (square) on a piece of itself, and double the aforementioned piece is cut in extreme and mean ratio, then the greater piece is the remaining part of the original straight line.
- (Which is) the very thing it was required to show.
∎
Thank you to the contributors under CC BY-SA 4.0! 

- Github:
-

- non-Github:
- @Fitzpatrick
References
Adapted from (subject to copyright, with kind permission)
- Fitzpatrick, Richard: Euclid's "Elements of Geometry"
