And when a straight line stood upon (another) straight line makes adjacent angles (which are) equal1 to one another, each of the equal angles is a right angle, and the former straight line is called a perpendicular to that upon which it stands.
When one segment \(\overline{AC}\) stands on another segment \(\overline{DB}\) such that the angles \(\angle{BAC}\) and \(\angle{CAD}\) are congruent, then both angles are called right angles, and their legs (e.g. segments, straight lines or rays) are called perpendicular to each other.
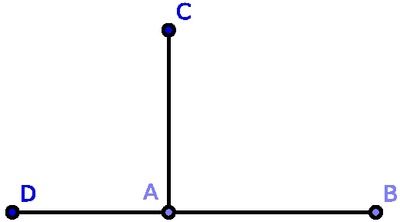
For any two perpendicular segments \(\overline{AC}\) and \(\overline{DB}\) we write \(\overline{AC}\perp\overline{DB}\).
Corollaries: 1
Axioms: 1 2
Corollaries: 3 4 5 6 7 8 9 10 11 12
Definitions: 13 14 15 16 17 18 19 20 21 22 23 24 25 26
Lemmas: 27 28
Parts: 29
Problems: 30
Proofs: 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 101 102 103 104 105 106 107 108 109 110 111 112 113 114 115 116 117 118 119 120 121 122 123 124 125 126 127 128 129 130 131 132 133 134 135 136
Propositions: 137 138 139 140 141 142 143 144 145 146 147 148 149 150 151 152 153 154 155 156 157 158 159 160 161 162 163 164 165 166 167 168 169 170 171 172 173
Sections: 174
Solutions: 175 176
The word "equal" is an intuitive definition used by Euclid about 2,200 years ago. In modern mathematics, one would first define what "equal" angles actually mean. This could, for instance, be accomplished by defining the operation counter-clockwise rotation of a given segment \(\overline{BA}\) around a fixed point \(A\) and the traditional degree apportionment of a full rotation (identified with the perigon $= 360^\circ$), which was already introduced by the Ancient Babylonians. Two angles would be equal if they have the same portion of the perigon, measured in degrees. Another possibility is to talk about congruence. ↩